Computational Boundary Condition (the Interpath Relation) for Spatially-Discrete Traveling-Wave Modulated Electromagnetic Struc

OVERVIEW

• Reduces the computational domain to a single stixel
• Reduces costs by derivation of the interpath relation boundary condition

BACKGROUND

Traveling-wave modulation electromagnetic structures play a pivotal role in the field of metamaterials by facilitating the creation of dynamic metamaterials, allowing real-time adjustments and adaptability to changing environmental conditions or desired functionalities. This modulation provides unique features that enable precise control over electromagnetic waves by manipulating their propagation characteristics, such as frequency, phase, and amplitude. This precision paves the way for transformative advancements in various fields that rely on electromagnetic wave manipulation. Traveling-wave modulation electromagnetic structures provide unique electromagnetic features and can be applied to amplification, frequency conversion, breaking reciprocity, and beam steering. While accurate simulation of the structures is critical to the design and can often be analyzed as continuous structure, spatially discrete structures yield more accurate models. However, simulation of spatially discrete structures can be costly due to the space-time dependency of the unit cells. As such, a need exists for simulations which are more cost efficient.

INNOVATION

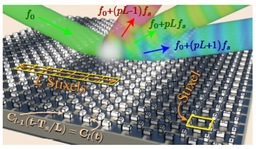
Researchers have developed a procedure for incorporating a novel boundary condition referred to as the "interpath relation" into existing frequency-domain computational electromagnetic solvers. The method can be used to simulate spatially-discrete traveling-wave modulation (SD-TWM) of electromagnetic structures, where SD-TWM structures are periodic in both space and time. Each spatial period of a SD-TWM structure consists of a number (N) of sub-cells known as "stixels", or space-time pixels. The time-variation of each stixel can be delayed with respect to the previous stixel by a time delay of T/N, where T is the temporal period of the modulation. The procedure therefore employs the interpath relation to reduce the computational domain to a single stixel. Compared to traditional computational methods, this approach reduces the number of required unknowns by the number of stixels contained within a spatial period. As that number grows, SD-TWM approaches continuous traveling-wave modulation. Therefore, the reduction in unknowns is proves meaningful for the simulation of continuous traveling-wave modulated structures. The derivation of the interpath relation boundary condition also reduces computational costs.